Potential energy is a fundamental concept in physics, representing the stored energy in an object due to its position, configuration, or state.
Understanding how to calculate potential energy is crucial for analyzing various physical systems, from simple mechanics to complex engineering structures.
Definition Of Potential Energy
Potential energy (PE) is the energy possessed by an object because of its position relative to other objects, internal stresses, electric charge, or other factors.
Definition Of Potential Energy
It is a form of mechanical energy, alongside kinetic energy, and plays a vital role in the conservation of energy within a system.
How to Calculate Potential Energy Using Our Potential Energy Calculator
Calculating potential energy is now easier than ever with the Potential Energy Calculator at PotentialEnergyCalculator.org.
Whether you’re a student or a professional, this tool simplifies the process and delivers accurate results in seconds—without doing manual calculations.
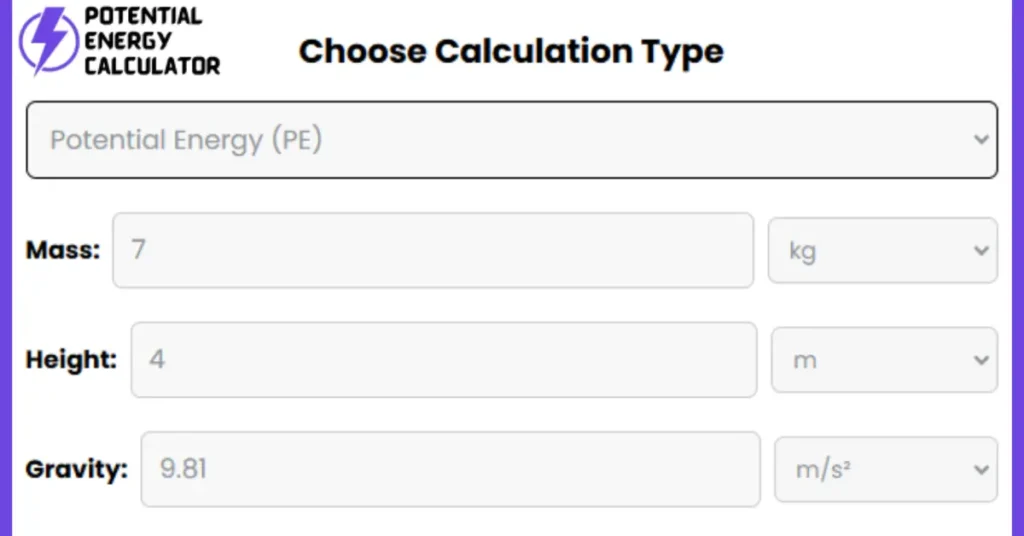
Here’s how to use it:
Step-by-Step Guide
1. Choose What to Calculate
- Visit the Potential Energy Calculator to access the calculator.
- Use the dropdown menu labeled “Choose Calculation Type”.
- Select from:
- Potential Energy (PE)
- Mass (m)
- Height (h)
- Gravity (g)
2. Enter the Required Values
- For PE, enter:
- Mass (e.g., 5 kg) with selectable units like kg, g, lb
- Height (e.g., 3 m) with units like m, ft, in
- Gravity (e.g., 9.81 m/s² by default)
- The form will automatically adjust based on the type of calculation you choose.
3. Click “Calculate”
- Once your values are filled, press the “Calculate” button.
- The result will appear instantly with multiple unit conversions, such as Joules, kilojoules, foot-pounds, and calories.
4. Optional: Share or Reset
- Use the “Share Results” button to copy or send a unique link containing your input data and results.
- Click “Reset” to clear all inputs and start fresh.
Types of Potential Energy
- Gravitational Potential Energy: Energy due to an object’s position in a gravitational field.
- Elastic Potential Energy: Energy stored in elastic materials as the result of their stretching or compressing.
- Electric Potential Energy: Energy due to the position of charged particles in an electric field.
- Chemical Potential Energy: Energy stored within chemical bonds.
- Nuclear Potential Energy: Energy stored within the nucleus of atoms.
Each type of potential energy has its own unique formula and calculation method, which we will discuss here in detail.
1. Gravitational Potential Energy (GPE)
Gravitational potential energy is the energy an object possesses due to its position in a gravitational field. The higher an object is positioned, the greater its gravitational potential energy.
Definition Of Gravitational Potential Energy

Formula for Gravitational Potential Energy
The formula to calculate gravitational potential energy is:
$$ PE=m×g×h $$
Where:
- m = mass of the object (in kilograms)
- g = acceleration due to gravity (approximately 9.81 m/s² on Earth)
- h = height above the reference point (in meters)
Example Calculation
Consider a 10 kg object lifted to a height of 5 meters.
$$ \text{PE} = 10 \, \text{kg} \times 9.8 \, \text{m/s}^2 \times 5 \, \text{m} = 490J \ $$
Thus, the gravitational potential energy is 490 joules.
You can also calculate Gravitational Potential Energy Online and instantly by using our Gravitational Potential Energy calculator.
Factors Affecting Gravitational Potential Energy
- Mass (m): Greater mass results in higher potential energy.
- Height (h): Higher elevation increases potential energy.
- Gravitational Acceleration (g): Varies with location (e.g., different planets).
Practical Applications
- Hydroelectric Power: Water stored at height possesses gravitational potential energy, which is converted into electricity.
- Roller Coasters: Carts at elevated tracks have high potential energy, transforming into kinetic energy during descent.
2. Elastic Potential Energy
Elastic potential energy is stored in objects that can be stretched or compressed, such as springs and rubber bands. This energy is released when the object returns to its original shape.
Definition Of Elastic Potential Energy

Formula for Elastic Potential Energy
The formula to calculate elastic potential energy is:
$$ \text{PE} = \frac{1}{2} \times k \times x^2 $$
Where:
- k = spring constant (in Newtons per meter)
- x = displacement from the equilibrium position (in meters)
Example Calculation
Consider a spring with a spring constant of 200 N/m compressed by 0.1 meters.
$$ \text{PE} = \frac{1}{2} \times 200 \, \text{N/m} \times (0.1 \, \text{m})^2 = 1 \, \text{J} $$
Thus, the elastic potential energy is 1 joule.
You can also calculate Elastic Potential Energy Online and instantly by using our Free Elastic Potential Energy calculator.
Factors Affecting Elastic Potential Energy
- Spring Constant (k): Stiffer springs (higher k) store more energy.
- Displacement (x): Greater deformation results in higher energy storage.
Practical Applications
- Archery: Drawing a bow stores elastic potential energy, released to propel the arrow.
- Vehicle Suspension: Springs absorb shocks, storing and releasing energy to smooth the ride.
3. Electric Potential Energy
Electric potential energy arises from the position of charged particles in an electric field. Like charges repel, and opposite charges attract, influencing the potential energy within the system.
Definition Of Electric Potential Energy
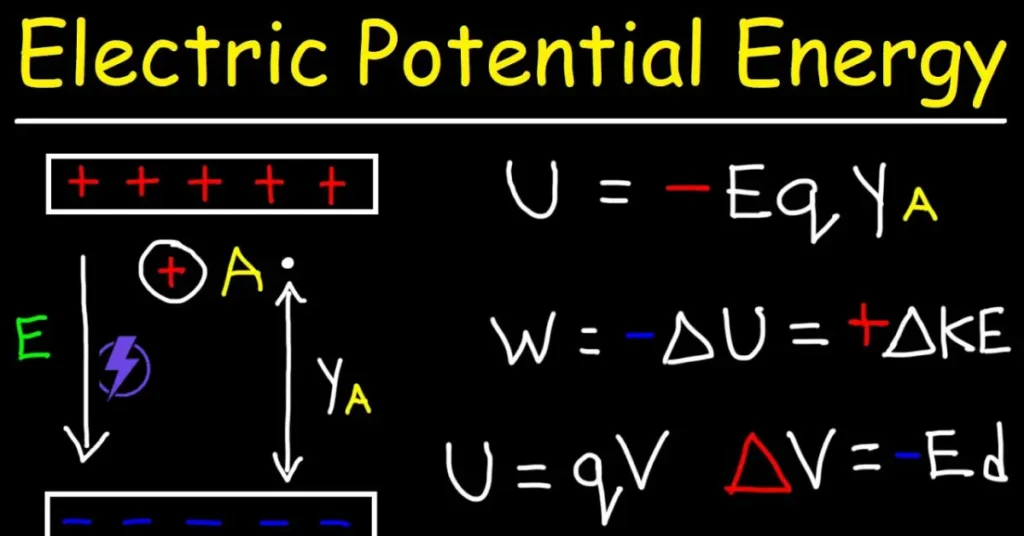
Formula for Electric Potential Energy
For two point charges, the electric potential energy is calculated as:
$$ \text{PE} = k_e \times \frac{q_1 \times q_2}{r} $$
Where:
r = distance between the charges (in meters)
$$ k_e = Coulomb’s \; constant \; (8.99 \times 10^9 \, \text{N m}^2/\text{C}^2) $$
$$ q_1, q_2 = magnitudes of the charges (in Coulombs) $$
Example Calculation (Electric Potential Energy)
Consider two charges:
- $$ q_1 = 2 \times 10^{-6} \, C $$
- $$ q_2 = 3 \times 10^{-6} \, C $$
- $$ Distance \, r = 0.05 \, m $$
Using:
$$ \text{PE} = k_e \cdot \frac{q_1 \cdot q_2}{r} $$
We get:
$$ PE = 0.058.99 \times 10^9 \times (2 \times 10^{-6} \times 3 \times 10^{-6}) $$
PE ≈ 1.08 Joules
This is the stored electric potential energy due to the positions of two charged particles.
You can also calculate Electric Potential Energy Online and instantly by using our free Electric Potential Energy calculator.
Comparison of Potential Energy Types
| Type | Formula | Stored In | Units |
|---|---|---|---|
| Gravitational | PE=m⋅g⋅h | Height and mass | Joules (J) |
| Elastic | $$ \text{PE} = \frac{1}{2} \times k \times x^2 $$ | Spring displacement | Joules (J) |
| Electric | $$ \text{PE} = k_e \cdot \frac{q_1 \cdot q_2}{r} $$ | Distance between electric charges | Joules (J) |
Common Units Used in Potential Energy Calculations
| Quantity | Unit (SI) | Symbol |
|---|---|---|
| Potential Energy | Joule | J |
| Mass | Kilogram | kg |
| Height / Displacement | Meter | m |
| Spring Constant | Newton/meter | N/m |
| Charge | Coulomb | C |
| Distance (electric) | Meter | m |
Solved Numerical Problems (Step-by-Step)
Problem 1: Gravitational PE
Q: What is the potential energy of a 12 kg object placed 3 meters high?
Solution:
PE = m × g × h
PE = 12 × 9.8 × 3 = 352.8 J
Problem 2: Elastic PE
Q: A spring with spring constant 250 N/m is compressed by 0.05 m. Calculate its PE.
Solution:
PE = ½ × k × x²
PE = ½ × 250 × (0.05)² = 0.3125 J
Problem 3: Electric PE
Q: Two charges q1 = 5 × 10−6 C, q2 = 2 × 10−6 C are placed 0.1 m apart. Find the potential energy (PE).
Solution:The formula for electric potential energy between two point charges is:
PE = ke × (q1 × q2) / r
Substituting the values:
PE = 8.99 × 109 × (5 × 10−6) × (2 × 10−6) / 0.1
PE = 8.99 × 109 × 10 × 10−12
PE = 0.899 J
FAQs
Yes, potential energy can be negative when the reference point is chosen above the object, such as in electric fields or gravitational wells.
The standard unit of potential energy in the International System (SI) is the Joule (J).
Mass, height, and gravitational acceleration affect gravitational PE. Higher mass or height increases the energy stored.
No, Potential energy is not the same as kinetic energy as Potential energy is stored energy due to position, while kinetic energy is energy due to motion. They often convert into each other.
In many systems, yes. For example, a falling object’s PE converts into kinetic energy as it gains speed.
Yes. Just use the gravitational acceleration of the specific planet in the formula PE=mgh.
No, It’s also found in rubber bands, bowstrings, trampolines, and any material that can stretch or compress and return to shape.
It’s a measure of a spring’s stiffness, given in Newtons per meter (N/m). A higher value means a stiffer spring.
Yes, If charges are alike, the electric potential energy is repulsive; if charges are opposite, it’s attractive.
Engineers, architects, physicists, and even sports scientists use PE formulas in design, analysis, and safety applications.

